Year :
2006
Title :
Mathematics (Core)
Exam :
WASSCE/WAEC MAY/JUNE
Paper 1 | Objectives
21 - 30 of 46 Questions
| # | Question | Ans |
|---|---|---|
| 21. |
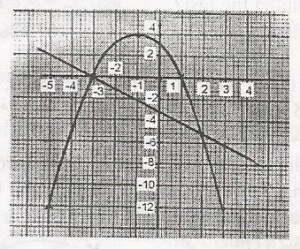 Which of these equations best describes the points of intersection of the curve and the line? A. \(x^2 + 5x - 6 = 0\) B. x2 - 5x - 6 = 0 C. x2 + x - 6 = 0 D. x2 - x - 6 = 0 Detailed SolutionThe roots are -3 and 1x = -3 and x = 1 y = (x + 3)(x - 1) = x2 - x + 3x - 3 y = x2 + x - 6 = 0 |
|
| 22. |
If loga270 - loga10 + loga \(\frac{1}{3}\) = 2, what is the value of a? A. 3 B. 4 C. 5 D. 6 Detailed Solutionloga270 - loga10 + loga\(\frac{1}{3}\) = 2loga270 + loga\(\frac{1}{3}\) - loga10 = 2 loga(270\(\frac{\frac{1}{3}}{10}\)) = 2 loga9 = 2 aa = 9 a = \(\sqrt{9}\) = 3 |
|
| 23. |
A conical water-jug is 7cm in diameter and 6cm deep. find the volume of water it can hold. [Take \(\pi = \frac{22}{7}\)] A. 22cm2 B. 44cm3 C. 77cm3 D. 3082 Detailed SolutionVolume = \(\frac{1}{3} \pi r^2h = \frac{1}{3} \times \frac{22}{7} \times \frac{7}{2} \times \frac{7}{2} \times \frac{6}{1}\)V = \(\frac{6468}{84}\) = 77cm3 |
|
| 24. |
If p = [\(\frac{Q(R - T)}{15}\)]\(^ \frac{1}{3}\), make T the subject of the relation A. T = R + \(\frac{P^3}{15Q}\) B. T = R - \(\frac{15P^3}{Q}\) C. T = R + \(\frac{P^3}{15Q}\) D. T = 15R - \(\frac{Q}{P^3}\) Detailed SolutionCubic both sides; P3 = \(\frac{Q(R - T)}{15}\)(cross multiplication) Q(R - T) = 15P3 (divide both sides by Q); R - T = 15\(\frac{1}{Q}\) (subtract r from both sides) - T = \(\frac{15P^3}{Q - R}\) T = R - \(\frac{15P^3}{Q}\) |
|
| 25. |
A seller allows 20% discount for cash payment on the marked price of his goods. What is the ratio of the cash payment to the marked price A. 1:4 B. 1:5 C. 3:4 D. 4:5 Detailed Solution(1 - \(\frac{20}{100}\)) = cash payment fraction= \(\frac{4}{5}\)The ratio of the cash payment to the marked price = 4:5 |
|
| 26. |
The wheel of a tractor has a diameter 1.4m. What distance does it cover in 100 complete revolutions? [Take \(\pi = \frac{22}{7}\)] A. 140m B. 220m C. 280m D. 440m Detailed Solutionradius = \(\frac{diameter}{2}\) = \(\frac{1.4}{2}\)= 0.7m circumference = 2\(\pi r = 2 \times \frac{22}{7} \times 0.7m = 4.4m\) distance covered = 4.4m x 100 = 440m |
|
| 27. |
If p = {1, 3, 5, 7, 9} and Q = {2, 4, 6, 8, 10} are subsets of a universal set. U = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10}. What are the elements of P1 \(\cap\) Q1? A. {1, 3, 5, 7, 9} B. [2, 4, 6, 8, 10} C. \(\varnothing\) D. {1, 2, 6, 8, 10} Detailed SolutionP1 = {2, 4, 6, 8, 10}; {1, 3, 5, 7, 9}P1 \(\cap\) q1 = {} or \(\varnothing\) |
|
| 28. |
The values of three angles at a point are 3y - 45o, y + 25o and yo . Find the value of y. A. 40o B. 58o C. 68o D. 76o Detailed Solution(3y - 450 + (y + 25) + t = 360o(angles at a point)3y - 45 + y + 25 + y = 360 5y = 360 + 20 5y = 380 y = \(\frac{380}{5}\) = 76o |
|
| 29. |
A rectangular carpet 2.5m long and 2.4m wide covers 5% of a rectangular floor. Calculate the area of the floor A. 30m2 B. 57m2 C. 120m2 D. 225m2 Detailed SolutionArea of carpet = 2.5 x 2.4 = 6Let F represent the floor 6 = 5% of F 6 = \(\frac{5}{100}\) x F 5F = 6 x 100 F = 6 x \(\frac{100}{5}\) = 120m2 |
|
| 30. |
Which of the following is not a measure of centre tendency? A. mean B. mode C. range D. median |
C |
| 21. |
 Which of these equations best describes the points of intersection of the curve and the line? A. \(x^2 + 5x - 6 = 0\) B. x2 - 5x - 6 = 0 C. x2 + x - 6 = 0 D. x2 - x - 6 = 0 Detailed SolutionThe roots are -3 and 1x = -3 and x = 1 y = (x + 3)(x - 1) = x2 - x + 3x - 3 y = x2 + x - 6 = 0 |
|
| 22. |
If loga270 - loga10 + loga \(\frac{1}{3}\) = 2, what is the value of a? A. 3 B. 4 C. 5 D. 6 Detailed Solutionloga270 - loga10 + loga\(\frac{1}{3}\) = 2loga270 + loga\(\frac{1}{3}\) - loga10 = 2 loga(270\(\frac{\frac{1}{3}}{10}\)) = 2 loga9 = 2 aa = 9 a = \(\sqrt{9}\) = 3 |
|
| 23. |
A conical water-jug is 7cm in diameter and 6cm deep. find the volume of water it can hold. [Take \(\pi = \frac{22}{7}\)] A. 22cm2 B. 44cm3 C. 77cm3 D. 3082 Detailed SolutionVolume = \(\frac{1}{3} \pi r^2h = \frac{1}{3} \times \frac{22}{7} \times \frac{7}{2} \times \frac{7}{2} \times \frac{6}{1}\)V = \(\frac{6468}{84}\) = 77cm3 |
|
| 24. |
If p = [\(\frac{Q(R - T)}{15}\)]\(^ \frac{1}{3}\), make T the subject of the relation A. T = R + \(\frac{P^3}{15Q}\) B. T = R - \(\frac{15P^3}{Q}\) C. T = R + \(\frac{P^3}{15Q}\) D. T = 15R - \(\frac{Q}{P^3}\) Detailed SolutionCubic both sides; P3 = \(\frac{Q(R - T)}{15}\)(cross multiplication) Q(R - T) = 15P3 (divide both sides by Q); R - T = 15\(\frac{1}{Q}\) (subtract r from both sides) - T = \(\frac{15P^3}{Q - R}\) T = R - \(\frac{15P^3}{Q}\) |
|
| 25. |
A seller allows 20% discount for cash payment on the marked price of his goods. What is the ratio of the cash payment to the marked price A. 1:4 B. 1:5 C. 3:4 D. 4:5 Detailed Solution(1 - \(\frac{20}{100}\)) = cash payment fraction= \(\frac{4}{5}\)The ratio of the cash payment to the marked price = 4:5 |
| 26. |
The wheel of a tractor has a diameter 1.4m. What distance does it cover in 100 complete revolutions? [Take \(\pi = \frac{22}{7}\)] A. 140m B. 220m C. 280m D. 440m Detailed Solutionradius = \(\frac{diameter}{2}\) = \(\frac{1.4}{2}\)= 0.7m circumference = 2\(\pi r = 2 \times \frac{22}{7} \times 0.7m = 4.4m\) distance covered = 4.4m x 100 = 440m |
|
| 27. |
If p = {1, 3, 5, 7, 9} and Q = {2, 4, 6, 8, 10} are subsets of a universal set. U = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10}. What are the elements of P1 \(\cap\) Q1? A. {1, 3, 5, 7, 9} B. [2, 4, 6, 8, 10} C. \(\varnothing\) D. {1, 2, 6, 8, 10} Detailed SolutionP1 = {2, 4, 6, 8, 10}; {1, 3, 5, 7, 9}P1 \(\cap\) q1 = {} or \(\varnothing\) |
|
| 28. |
The values of three angles at a point are 3y - 45o, y + 25o and yo . Find the value of y. A. 40o B. 58o C. 68o D. 76o Detailed Solution(3y - 450 + (y + 25) + t = 360o(angles at a point)3y - 45 + y + 25 + y = 360 5y = 360 + 20 5y = 380 y = \(\frac{380}{5}\) = 76o |
|
| 29. |
A rectangular carpet 2.5m long and 2.4m wide covers 5% of a rectangular floor. Calculate the area of the floor A. 30m2 B. 57m2 C. 120m2 D. 225m2 Detailed SolutionArea of carpet = 2.5 x 2.4 = 6Let F represent the floor 6 = 5% of F 6 = \(\frac{5}{100}\) x F 5F = 6 x 100 F = 6 x \(\frac{100}{5}\) = 120m2 |
|
| 30. |
Which of the following is not a measure of centre tendency? A. mean B. mode C. range D. median |
C |