Year :
2007
Title :
Mathematics (Core)
Exam :
WASSCE/WAEC MAY/JUNE
Paper 1 | Objectives
11 - 20 of 67 Questions
| # | Question | Ans |
|---|---|---|
| 11. |
If x =3, y = 2 and z = 4 what is the value of \(3x^{2}-2y+z\) A. 17 B. 27 C. 35 D. 71 Detailed Solution\(3x^{2}-2y+z=3(3)^{2}-2(2)+4=27-4+4=27\) |
|
| 12. |
Solve the simultaneous equation: x+y=2 and 3x-2y=1 A. x=2 and y=1 B. x=1 and y=1 C. x=1 and y=2 D. x=-1 and y=1 Detailed Solutionx + y = 2 --- eqn I3x - 2y = 1 --- eqn II Multiply eqn by 2 2x + 2y = 2 --- eqn III Subtract eqn II from eqnI 5x = 5 => x = 1 Substitute 1 for x in eqn 1 1 + y = 2 => y = 1 |
|
| 13. |
If \(8^{x+1}=\frac{1}{4}\), find x A. \(-\frac{5}{3}\) B. -1 C. \(-\frac{1}{3}\) D. \(\frac{1}{3}\) Detailed Solution\(8^{x+1}=\frac{1}{4}\\2^{3(x+1)}=2^{-2}\Rightarrow 3(x+1)=-2\Rightarrow 3x+3=-2\\ 3x=-5\Rightarrow x=\frac{5}{3}\) |
|
| 14. |
The area of a square field is 110.25m2. Find the cost of fencing it round at N75.00 per meter square A. N1,575.00 B. N3,150.00 C. N4,734.30 D. N8,268.75 Detailed SolutionN75.00 x 110.25 = N8,268.75 |
|
| 15. |
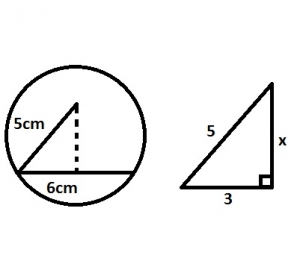
A chord of length 6cm is drawn in a circle of radius 5cm. Find the distance of the chord from the center of the circle A. 2.5cm B. 3.0cm C. 3.5cm D. 4.0cm Detailed Solution
 25-9=x^{2}\\ 16=x^{2}\Rightarrow x=4.0\) |
|
| 16. |
A sector of a circle of radius 14cm containing an angle 60o is folded to form a cone. Calculate the radius of the base of the cone. A. \(5\frac{1}{2}cm\) B. \(4\frac{2}{3}cm\) C. \(3\frac{1}{2}cm\) D. \(2\frac{1}{3}cm\) Detailed Solution\(r=\frac{\theta \times l}{360^{\circ }}\\r=?, \theta =60^{\circ }\), l = radius of the orginal sector of a circle = 14cm \(r=\frac{60\times 14}{360}=\frac{7}{3}=2\frac{1}{3}cm\) |
|
| 17. |
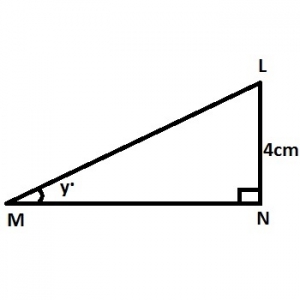 IN the diagram, |LN| = 4cm, LNM = 90o and tan y = 2/3. What is the area of the ∆LMN? A. 24cm2 B. 12cm2 C. 10cm2 D. 6cm2 Detailed Solution\(tan \hspace{1mm}y = \frac{opp}{adj}=\frac{2}{3}\Rightarrow \frac{2}{3}=\frac{LN}{MN}=\frac{4}{MN}\\ ∴ MN = \frac{12}{2}=6\\ Area \hspace{1mm}of \hspace{1mm}\Delta LMN = \frac{1}{2}\times MN\times LN=\frac{1}{2}\times 6\times 4=12cm\) |
|
| 18. |
Evaluate(111two)2 and leave your answer in base 2. A. 111001two B. 110001two C. 101001two D. 10010two |
B |
| 19. |
Find the LCM of 23 x 3 x 52, 2 x 32 x 5 and 23 x 33 x 5 A. 22 x 33 x 52 B. 23 x 33 x 5 C. 22 x 32 x 52 D. 23 x 33 x 52 Detailed Solution23 x 3 x 522 x 32 x 5 22 x 33 x 5 The L.C.M = 22 x 33 x 52 |
|
| 20. |
Simplify(0.3 x 105) \(\div\) (0.4 \(\times\) 107), leaving you answer in the standard form. A. 7.5 x 10-4 B. 7.5 x 10-3 C. 7.5 x 10-2 D. 7.5 x 10-1 Detailed Solution\(\frac{0.3 \times 10^5}{0.4 \times 10^7} = \frac{3 \times 10^{-1} \times 10^{5}}{4 \times 10^{-1} \times 10^7}\)\(\frac{3 x 10^4}{4 x 10^4}\) \(\frac{3}{4}\) x 104-6 = 0.75 x 10-2 = 7.5 x 10-3 |
| 11. |
If x =3, y = 2 and z = 4 what is the value of \(3x^{2}-2y+z\) A. 17 B. 27 C. 35 D. 71 Detailed Solution\(3x^{2}-2y+z=3(3)^{2}-2(2)+4=27-4+4=27\) |
|
| 12. |
Solve the simultaneous equation: x+y=2 and 3x-2y=1 A. x=2 and y=1 B. x=1 and y=1 C. x=1 and y=2 D. x=-1 and y=1 Detailed Solutionx + y = 2 --- eqn I3x - 2y = 1 --- eqn II Multiply eqn by 2 2x + 2y = 2 --- eqn III Subtract eqn II from eqnI 5x = 5 => x = 1 Substitute 1 for x in eqn 1 1 + y = 2 => y = 1 |
|
| 13. |
If \(8^{x+1}=\frac{1}{4}\), find x A. \(-\frac{5}{3}\) B. -1 C. \(-\frac{1}{3}\) D. \(\frac{1}{3}\) Detailed Solution\(8^{x+1}=\frac{1}{4}\\2^{3(x+1)}=2^{-2}\Rightarrow 3(x+1)=-2\Rightarrow 3x+3=-2\\ 3x=-5\Rightarrow x=\frac{5}{3}\) |
|
| 14. |
The area of a square field is 110.25m2. Find the cost of fencing it round at N75.00 per meter square A. N1,575.00 B. N3,150.00 C. N4,734.30 D. N8,268.75 Detailed SolutionN75.00 x 110.25 = N8,268.75 |
|
| 15. |
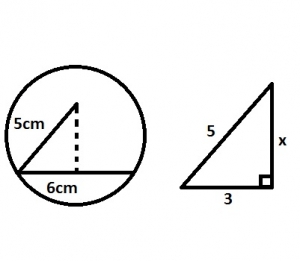
A chord of length 6cm is drawn in a circle of radius 5cm. Find the distance of the chord from the center of the circle A. 2.5cm B. 3.0cm C. 3.5cm D. 4.0cm Detailed Solution
 25-9=x^{2}\\ 16=x^{2}\Rightarrow x=4.0\) |
| 16. |
A sector of a circle of radius 14cm containing an angle 60o is folded to form a cone. Calculate the radius of the base of the cone. A. \(5\frac{1}{2}cm\) B. \(4\frac{2}{3}cm\) C. \(3\frac{1}{2}cm\) D. \(2\frac{1}{3}cm\) Detailed Solution\(r=\frac{\theta \times l}{360^{\circ }}\\r=?, \theta =60^{\circ }\), l = radius of the orginal sector of a circle = 14cm \(r=\frac{60\times 14}{360}=\frac{7}{3}=2\frac{1}{3}cm\) |
|
| 17. |
 IN the diagram, |LN| = 4cm, LNM = 90o and tan y = 2/3. What is the area of the ∆LMN? A. 24cm2 B. 12cm2 C. 10cm2 D. 6cm2 Detailed Solution\(tan \hspace{1mm}y = \frac{opp}{adj}=\frac{2}{3}\Rightarrow \frac{2}{3}=\frac{LN}{MN}=\frac{4}{MN}\\ ∴ MN = \frac{12}{2}=6\\ Area \hspace{1mm}of \hspace{1mm}\Delta LMN = \frac{1}{2}\times MN\times LN=\frac{1}{2}\times 6\times 4=12cm\) |
|
| 18. |
Evaluate(111two)2 and leave your answer in base 2. A. 111001two B. 110001two C. 101001two D. 10010two |
B |
| 19. |
Find the LCM of 23 x 3 x 52, 2 x 32 x 5 and 23 x 33 x 5 A. 22 x 33 x 52 B. 23 x 33 x 5 C. 22 x 32 x 52 D. 23 x 33 x 52 Detailed Solution23 x 3 x 522 x 32 x 5 22 x 33 x 5 The L.C.M = 22 x 33 x 52 |
|
| 20. |
Simplify(0.3 x 105) \(\div\) (0.4 \(\times\) 107), leaving you answer in the standard form. A. 7.5 x 10-4 B. 7.5 x 10-3 C. 7.5 x 10-2 D. 7.5 x 10-1 Detailed Solution\(\frac{0.3 \times 10^5}{0.4 \times 10^7} = \frac{3 \times 10^{-1} \times 10^{5}}{4 \times 10^{-1} \times 10^7}\)\(\frac{3 x 10^4}{4 x 10^4}\) \(\frac{3}{4}\) x 104-6 = 0.75 x 10-2 = 7.5 x 10-3 |