Mathematics (Core)
Paper 1 | Objectives | 80 Questions
JAMB Exam
Year: 2019
Level: SHS
Time:
Type: Question Paper
Answers provided
FREE
No description provided
Feedbacks
This paper is yet to be rated
Paper 1 | Objectives | 80 Questions
JAMB Exam
Year: 2019
Level: SHS
Time:
Type: Question Paper
Answers provided
No description provided
This paper is yet to be rated
| # | Question | Ans |
|---|---|---|
| 1. |
Make q the subject of the formula in the equation \(\frac{mn}{a^2} - \frac{pq}{b^2} = 1\) A. \(q = \frac{b^2(mn - a^2)}{a^2 p}\) B. \(q = \frac{m^2 n - a^2}{p^2}\) C. \(q = \frac{mn - 2b^2}{a^2}\) D. \(q = \frac{b^2 (n^2 - ma^2)}{n}\) Detailed Solution\(\frac{mn}{a^2} - \frac{pq}{b^2} = 1\)\(\frac{mn}{a^2} - 1 = \frac{pq}{b^2}\) \(\frac{mn - a^2}{a^2} = \frac{pq}{b^2}\) \(pq = \frac{b^2 (mn - a^2)}{a^2}\) \(q = \frac{b^2(mn - a^2)}{a^2 p}\) There is an explanation video available below. |
|
| 2. |
The angle of elevation of the top of a tree from a point on the ground 60m away from the foot of the tree is 78°. Find the height of the tree correct to the nearest whole number. A. 148m B. 382m C. 282m D. 248m Detailed Solution \(\tan 78 = \frac{h}{60}\) \(h = 60 \tan 78\) \(h = 60 \times 4.705 = 282.27m\) \(\approxeq\) 282m to the nearest whole number. There is an explanation video available below. |
|
| 3. |
A binary operation \(\otimes\) is defined by \(m \otimes n = mn + m - n\) on the set of real numbers, for all m, n \(\in\) R. Find the value of 3 \(\otimes\) (2 \(\otimes\) 4). A. 6 B. 25 C. 15 D. 18 Detailed Solution\(m \otimes n = mn + m - n\)3 \(\otimes\) (2 \(\otimes\) 4) 2 \(\otimes\) 4 = 2(4) + 2 - 4 = 6 3 \(otimes\) 6 = 3(6) + 3 - 6 = 15 There is an explanation video available below. |
|
| 4. |
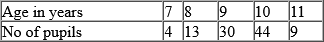 Table:The table above shows the number of pupils in a class with respect to their ages. If a pie chart is constructed to represent the age, the angle corresponding to 8 years old is A. 48.6° B. 56.3° C. 46.8° D. 13° Detailed SolutionTotal number of pupils : 4 + 13 + 30 + 44 + 9 = 100The number of 8 - year olds = 13 The angle represented by the 8-year olds on the pie chart = \(\frac{13}{100} \times 360°\) = 46.8° There is an explanation video available below. |
|
| 5. |
In a class of 50 students, 40 students offered Physics and 30 offered Biology. How many offered both Physics and Biology? A. 42 B. 20 C. 70 D. 54 Detailed Solutionn(Total) = 50n(Physics) = 40 n(Biology) = 30 Let n(Physics and Biology) = x n(Physics only) = 40 -x n(Biology only) = 30 - x 40 - x + 30 - x + x = 50 70 - x = 50 x = 20 There is an explanation video available below. |
|
| 6. |
Rationalize \(\frac{\sqrt{2} + \sqrt{3}}{\sqrt{2} - \sqrt{3}}\) A. \(-5 - 2\sqrt{6}\) B. \(-5 + 3\sqrt{2}\) C. \(5 - 2\sqrt{3}\) D. \(5 + 2\sqrt{6}\) Detailed Solution\(\frac{\sqrt{2} + \sqrt{3}}{\sqrt{2} - \sqrt{3}}\)= \((\frac{\sqrt{2} + \sqrt{3}}{\sqrt{2} - \sqrt{3}})(\frac{\sqrt{2} + \sqrt{3}}{\sqrt{2} + \sqrt{3}})\) = \(\frac{2 + \sqrt{6} + \sqrt{6} + 3}{2 - \sqrt{6} + \sqrt{6} - 3}\) = \(\frac{5 + 2\sqrt{6}}{-1}\) = \(- 5 - 2\sqrt{6}\) There is an explanation video available below. |
|
| 7. |
OPEN_PHOTO Find the length of the chord |AB| in the diagram shown above. A. 4.2 cm B. 4.3 cm C. 3.2 cm D. 3.4 cm Detailed SolutionLength of chord = \(2r \sin (\frac{\theta}{2})\)= \(2(3) \sin (\frac{68}{2})\) = \(6 \sin 34\) = \(6 \times 0.559\) = 3.354 cm \(\approxeq\) 3.4 cm There is an explanation video available below. |
|
| 8. |
Given \(\sin 58° = \cos p°\), find p. A. 48° B. 58° C. 32° D. 52° Detailed Solution\(\sin \theta = \cos (90 - \theta)\)\(\sin \theta = \cos (90 - 58)\) = \(\cos 32\) There is an explanation video available below. |
|
| 9. |
\(\frac{\frac{2}{3} \div \frac{4}{5}}{\frac{1}{4} + \frac{3}{5} - \frac{1}{3}}\) A. \(\frac{31}{50}\) B. \(\frac{20}{31}\) C. \(\frac{31}{20}\) D. \(\frac{50}{31}\) Detailed Solution\(\frac{\frac{2}{3} \div \frac{4}{5}}{\frac{1}{4} + \frac{3}{5} - \frac{1}{3}}\)\(\frac{2}{3} \div \frac{4}{5} = \frac{2}{3} \times \frac{5}{4}\) = \(\frac{5}{6}\) \(\frac{1}{4} + \frac{3}{5} - \frac{1}{3} = \frac{15 + 36 - 20}{60}\) = \(\frac{31}{60}\) \(\therefore \frac{\frac{2}{3} \div \frac{4}{5}}{\frac{1}{4} + \frac{3}{5} - \frac{1}{3}} = \frac{5}{6} \div \frac{31}{60}\) = \(\frac{5}{6} \times \frac{60}{31}\) = \(\frac{50}{31}\) There is an explanation video available below. |
|
| 10. |
If \(6x^3 + 2x^2 - 5x + 1\) divides \(x^2 - x - 1\), find the remainder. A. 9x + 9 B. 2x + 6 C. 6x + 8 D. 5x - 3 |
|
| 11. |
If a fair coin is tossed 3 times, what is the probability of getting at least two heads? A. \(\frac{2}{3}\) B. \(\frac{4}{5}\) C. \(\frac{2}{5}\) D. \(\frac{1}{2}\) Detailed SolutionThe outcomes are {HHH, HHT, HTT, HTH, THH, THT, TTH, TTT}P(at least two heads) = \(\frac{4}{8}\) = \(\frac{1}{2}\) There is an explanation video available below. |
|
| 12. |
In how many ways can the word MATHEMATICIAN be arranged? A. 6794800 ways B. 2664910 ways C. 6227020800 ways D. 129729600 ways Detailed SolutionMATHEMATICIAN = 13 letters with 2M, 3A, 2T, 2I.Hence, the word MATHEMATICIAN can be arranged in \(\frac{13!}{2! 3! 2! 2!}\) = 129729600 ways There is an explanation video available below. |
|
| 13. |
Given matrix M = \(\begin{vmatrix} -2 & 0 & 4 \\ 0 & -1 & 6 \\ 5 & 6 & 3 \end{vmatrix}\), find \(M^{T} + 2M\) A. \(\begin{vmatrix} -4 & 2 & 1\\ 6 & 0 & 5 \\ 0 & 6 & 2 \end{vmatrix}\) B. \(\begin{vmatrix} -6 & 0 & 13\\ 0 & -3 & 18 \\ 14 & 18 & 9 \end{vmatrix}\) C. \(\begin{vmatrix} 5 & 2 & 6 \\ 0 & 1 & 1\\ 3 & 4 & -7 \end{vmatrix}\) D. \(\begin{vmatrix} -4 & 0 & 8 \\ 0 & -2 & -16 \\ 10 & 12 & 6 \end{vmatrix}\) Detailed SolutionM = \(\begin{vmatrix} -2 & 0 & 4 \\ 0 & -1 & 6 \\ 5 & 6 & 3 \end{vmatrix}\)M\(^{T}\) = \(\begin{vmatrix} -2 & 0 & 5 \\ 0 & -1 & 6\\ 4 & 6 & 3 \end{vmatrix}\) 2M = \(\begin{vmatrix} -4 & 0 & 8\\ 0 & -2 & 12\\ 10 & 12 & 6\end{vmatrix}\) M\(^T\) + 2M = \(\begin{vmatrix} -6 & 0 & 13 \\ 0 & -3 & 18 \\ 14 & 18 & 9 \end{vmatrix}\) There is an explanation video available below. |
|
| 14. |
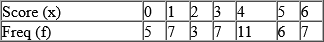 Table:Find the mean of the data. A. 3.26 B. 4.91 C. 6.57 D. 3.0 Detailed SolutionMean = \(\frac{\sum fx}{\sum f}\)= \(\frac{150}{46}\) = 3.26 There is an explanation video available below. |
|
| 15. |
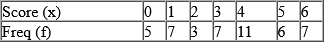 Table:Find the variance A. 3.42 B. 4.69 C. 4.85 D. 3.72 Detailed SolutionVariance = \(\frac{\sum f(x - \bar{x})}{\sum f}\)= \(\frac{170.888}{46}\) = 3.72 There is an explanation video available below. |
|
| 16. |
The locus of a point which moves so that it is equidistant from two intersecting straight lines is the A. bisector of the two lines B. line parallel to the two lines C. angle bisector of the two lines D. perpendicular bisector of the two lines Detailed SolutionThe locus of a points equidistant from two intersecting straight lines is a pair of bisectors that bisect the angles formed by the two intersecting lines.There is an explanation video available below. |
Preview displays only 16 out of the 80 Questions
