Paper Info

Year :

1999

Title :

Mathematics (Core)

Exam :

WASSCE/WAEC MAY/JUNE

Paper 1 | Objectives

41 - 43 of 43 Questions

#	Question	Ans
41.	The sides of a right angle triangle in ascending order of magnitude are 8cm, (x-2)cm and x cm. Find x A. 16 B. 17 C. 34 D. 90 Show Content Detailed Solution \(8^2 + (x - 2)^2 = x^2\\ 64 + x^2 - 4x + 4 = x^{2}\\ x^2 - 4x + 68 = x^2\\ -4x = -68\\ -x = \frac{-68}{4} = 17\)	B
42.	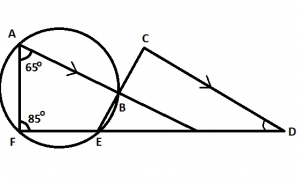In the diagram, CDE is a triangle, ABEF is cyclic quadrilateral, AB\|\|CD, ∠BAF = 65^o and ∠AFE = 85^o calculate ∠CDE A. 20^o B. 30^o C. 50^o D. 65^o	B
43.	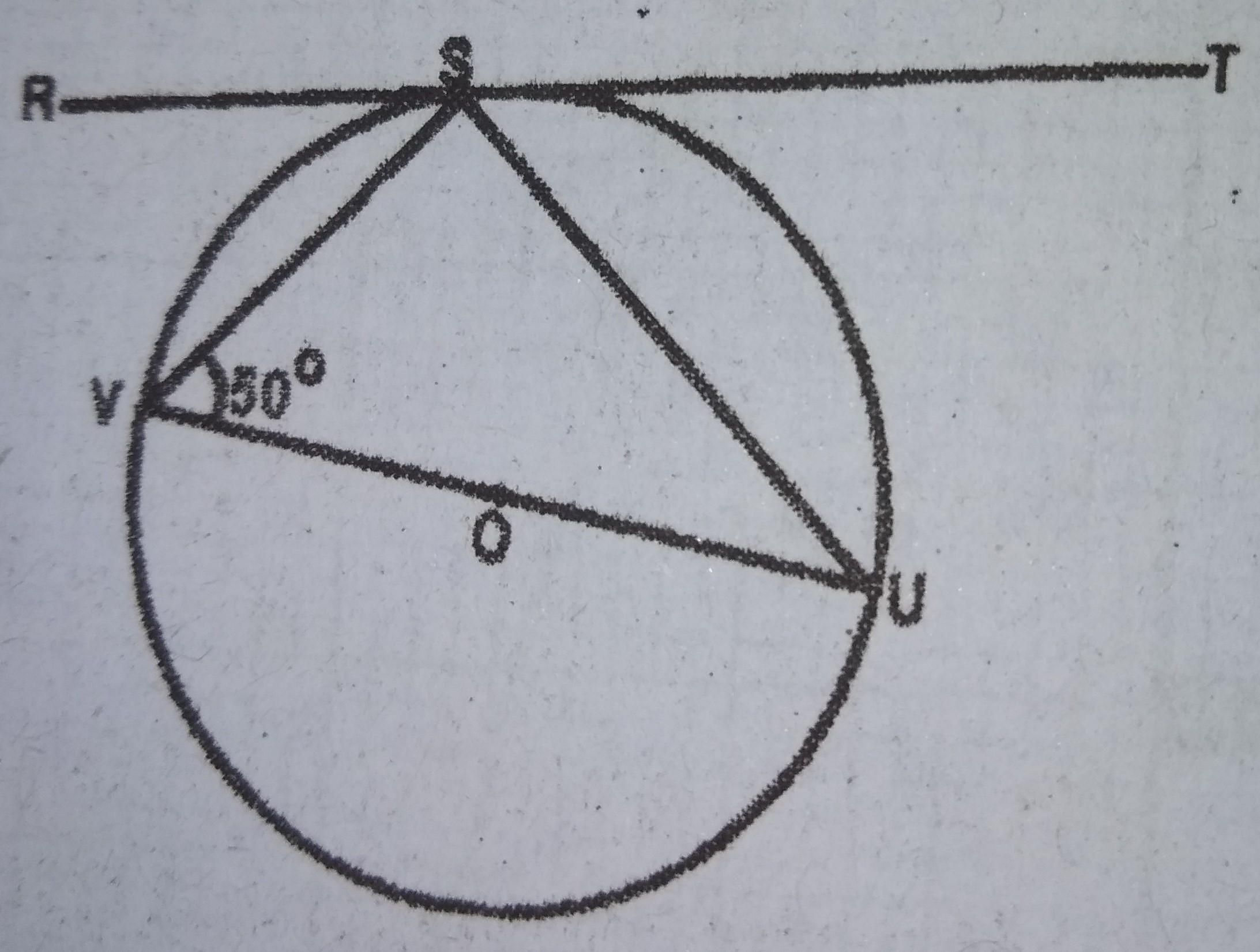In the diagram above, RST is a tangent to circle VSU center O ∠SVU = 50° and UV is a diameter. Calculate ∠RSV. A. 90° B. 50° C. 45° D. 40° Show Content Detailed Solution < VSU = 90° (angle in a semi-circle) \(\therefore\) < SUV = 180° - 90° - 50° = 40° < RSV = 40° (angle which chord makes tangent = angle in alternate segment)	D

41.	The sides of a right angle triangle in ascending order of magnitude are 8cm, (x-2)cm and x cm. Find x A. 16 B. 17 C. 34 D. 90 Show Content Detailed Solution \(8^2 + (x - 2)^2 = x^2\\ 64 + x^2 - 4x + 4 = x^{2}\\ x^2 - 4x + 68 = x^2\\ -4x = -68\\ -x = \frac{-68}{4} = 17\)	B
42.	In the diagram, CDE is a triangle, ABEF is cyclic quadrilateral, AB\|\|CD, ∠BAF = 65^o and ∠AFE = 85^o calculate ∠CDE A. 20^o B. 30^o C. 50^o D. 65^o	B

43.

In the diagram above, RST is a tangent to circle VSU center O ∠SVU = 50° and UV is a diameter. Calculate ∠RSV.

A. 90°

B. 50°

C. 45°

D. 40°

Detailed Solution

< VSU = 90° (angle in a semi-circle)
\(\therefore\) < SUV = 180° - 90° - 50°
= 40°
< RSV = 40° (angle which chord makes tangent = angle in alternate segment)