Year :
1989
Title :
Mathematics (Core)
Exam :
WASSCE/WAEC MAY/JUNE
Paper 1 | Objectives
31 - 40 of 48 Questions
| # | Question | Ans |
|---|---|---|
| 31. |
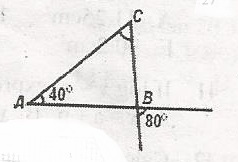 In the diagram above, what is the size of angle ACB? A. 40o B. 50o C. 60o D. 80o E. 100o Detailed Solution< ABC = 80° (alternate angles)< ACB = 180° - (80° + 40°) = 60° |
|
| 32. |
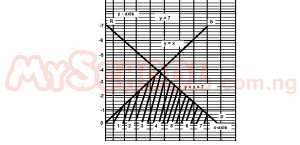 With reference to the graph above, which f the following solution sets is represented by ΔOPQ? A. y ≥ x, y ≤ 0, y + x ≥ 7 B. y ≤ x, y ≥ 0, y + x ≤ 7 C. y ≥ x, y ≥ 0, y + x ≤ 7 D. y ≤ x, y ≤ 0, y + x ≤ 7 E. y ≤ x, y ≥ 0, y + x ≥ 7 |
D |
| 33. |
Find the volume of a cone of radius 3.5cm and vertical height 12cm [Take π = 22/7] A. 3.4cm2 B. 15.5cm2 C. 21.0cm2 D. 42.0cm2 E. 154.0cm2 |
E |
| 34. |
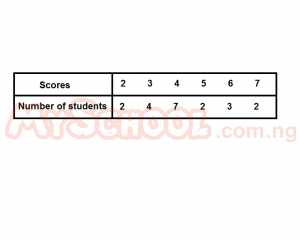 The table above gives the scores of a group of students in an English Language test A. 3/20 B. 1/5 C. 1/4 D. 3/10 E. 3/4 Detailed SolutionProbability = 5/20 = 1/4 |
|
| 35. |
The angle of a sector of a circle of diameter 8cm is 135° [Take x = 22/7]. A. 270 B. 350 C. 302 D. 430 Detailed SolutionArea of a sectorθπr2/360° = (135 / 360) X 3.142 X 16 X 16 = 301.6 ≈ 302cm2 |
|
| 36. |
 In the diagram above, O is the center of the circle QRT and PT is a tangent to the circle at T. Calculate the angle x A. 55o B. 40o C. 35o D. 25o E. 20o Detailed Solution∠QPT = xo = 180o - 125o - 35o = 20o |
|
| 37. |
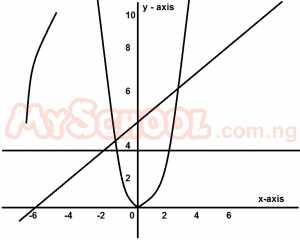 Which of the following gives the point of intersection of the graph y = x2 and y = x + 6 shown above? A. (0,6), (3,9) B. (-3, 0)(2,4) C. (-2,4)(3,9) D. (-2,3)(-3,2) E. (-2,2)(3,6) Detailed SolutionFrom the graph; when x = -2, y = 4(-2, 4) x = 3.0, y = 9; (3,9) (-2, 4)(3,9) |
|
| 38. |
A sector is cut off from a circle radius 8.2cm to form a cone, if the radius of the resulting cone is 3.5cm, calculate the curved surface area of the cone. [take π = 22/7] A. 11.25cm2 B. 12.83cm2 C. 22.0cm2 D. 67.2cm2 E. 90.2cm2 Detailed Solution r = 3.5 cm; l = 8.2 cm. Curved surface area = \(\pi rl\) = \(\frac{22}{7} \times \frac{7}{2} \times \frac{41}{5}\) = 90.2 cm\(^2\) |
|
| 39. |
If logax = p, express x in terms of a and p A. x = u + p B. x = a/p C. x = p D. x = ap E. x = ap Detailed Solution\(\log_{a} x = p\)\(x = a^p\) |
|
| 40. |
Calculate and correct to three significant figures, the length of an arc subtends an angle of 70o at the center of the circle radius 4cm. [Take π = 22/7] A. 2.44cm B. 4.89cm C. 9.78cm D. 25.1cm E. 50.3cm Detailed SolutionCircumference of circle = 2πr = 2 x 3.14 x 4= 25.1cm 360o = 25.1cm 70o = 25.1360 x 70 = 4.89 |
| 31. |
 In the diagram above, what is the size of angle ACB? A. 40o B. 50o C. 60o D. 80o E. 100o Detailed Solution< ABC = 80° (alternate angles)< ACB = 180° - (80° + 40°) = 60° |
|
| 32. |
 With reference to the graph above, which f the following solution sets is represented by ΔOPQ? A. y ≥ x, y ≤ 0, y + x ≥ 7 B. y ≤ x, y ≥ 0, y + x ≤ 7 C. y ≥ x, y ≥ 0, y + x ≤ 7 D. y ≤ x, y ≤ 0, y + x ≤ 7 E. y ≤ x, y ≥ 0, y + x ≥ 7 |
D |
| 33. |
Find the volume of a cone of radius 3.5cm and vertical height 12cm [Take π = 22/7] A. 3.4cm2 B. 15.5cm2 C. 21.0cm2 D. 42.0cm2 E. 154.0cm2 |
E |
| 34. |
 The table above gives the scores of a group of students in an English Language test A. 3/20 B. 1/5 C. 1/4 D. 3/10 E. 3/4 Detailed SolutionProbability = 5/20 = 1/4 |
|
| 35. |
The angle of a sector of a circle of diameter 8cm is 135° [Take x = 22/7]. A. 270 B. 350 C. 302 D. 430 Detailed SolutionArea of a sectorθπr2/360° = (135 / 360) X 3.142 X 16 X 16 = 301.6 ≈ 302cm2 |
| 36. |
 In the diagram above, O is the center of the circle QRT and PT is a tangent to the circle at T. Calculate the angle x A. 55o B. 40o C. 35o D. 25o E. 20o Detailed Solution∠QPT = xo = 180o - 125o - 35o = 20o |
|
| 37. |
 Which of the following gives the point of intersection of the graph y = x2 and y = x + 6 shown above? A. (0,6), (3,9) B. (-3, 0)(2,4) C. (-2,4)(3,9) D. (-2,3)(-3,2) E. (-2,2)(3,6) Detailed SolutionFrom the graph; when x = -2, y = 4(-2, 4) x = 3.0, y = 9; (3,9) (-2, 4)(3,9) |
|
| 38. |
A sector is cut off from a circle radius 8.2cm to form a cone, if the radius of the resulting cone is 3.5cm, calculate the curved surface area of the cone. [take π = 22/7] A. 11.25cm2 B. 12.83cm2 C. 22.0cm2 D. 67.2cm2 E. 90.2cm2 Detailed Solution r = 3.5 cm; l = 8.2 cm. Curved surface area = \(\pi rl\) = \(\frac{22}{7} \times \frac{7}{2} \times \frac{41}{5}\) = 90.2 cm\(^2\) |
|
| 39. |
If logax = p, express x in terms of a and p A. x = u + p B. x = a/p C. x = p D. x = ap E. x = ap Detailed Solution\(\log_{a} x = p\)\(x = a^p\) |
|
| 40. |
Calculate and correct to three significant figures, the length of an arc subtends an angle of 70o at the center of the circle radius 4cm. [Take π = 22/7] A. 2.44cm B. 4.89cm C. 9.78cm D. 25.1cm E. 50.3cm Detailed SolutionCircumference of circle = 2πr = 2 x 3.14 x 4= 25.1cm 360o = 25.1cm 70o = 25.1360 x 70 = 4.89 |