Year :
2008
Title :
Mathematics (Core)
Exam :
JAMB Exam
Paper 1 | Objectives
41 - 49 of 49 Questions
| # | Question | Ans |
|---|---|---|
| 41. |
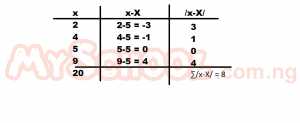
Find the mean deviation of 2, 4, 5, and 9 A. 1 B. 2 C. 5 D. 7 Detailed Solution
= 5 mean deviation = 8/4 = 2 There is an explanation video available below. |
|
| 42. |
In how many ways can the letters of the word ACCEPTANCE be arranged? A. 10! / (2!2!3!) B. 10! / ( 2!3!) C. 10! / (2!2!) D. 10! Detailed SolutionACCEPTANCE = 10 LettersA = 2 letters C = 3 letters E = 2 letters Can be arranged in 10! / (2!3!2!) ways There is an explanation video available below. |
|
| 43. |
Find the number of ways of selecting 6 out of 10 subjects for an examination A. 128 B. 216 C. 215 D. 210 Detailed Solution\(^{10}C_6 = \frac{10!}{(10-6)!6!}\\=\frac{10!}{4!6!}\\ =\frac{10\times 9\times 8 \times 7 \times 6!}{4\times 3\times 2\times 1 \times 6!}\\ =210\) There is an explanation video available below. |
|
| 44. |
The probability of picking a letter T from the word OBSTRUCTION is? A. 1/11 B. 2/11 C. 3/11 D. 4/11 Detailed SolutionOBSTRUCTIONTotal possible outcome = 11 Number of chance of getting T = 2 P(picking T) = 2/11 There is an explanation video available below. |
|
| 45. |
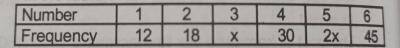 The result of rolling a fair die 150 times is as summarized in the table above. What is the probability of obtaining a 5? A. 3/10 B. 1/5 C. 1/6 D. 1/10 Detailed SolutionTotal possible outcome12+18+x+30+2x+45 = 105+3x ∴105+3x = 150 3x = 150-105 3x = 45 x = 15 P(obtaining 5) = \(\frac{2x}{(105+3x)}But x= 15\\ =\frac{2(15)}{(105+3(15))}\\ =\frac{30}{(105+45)}\\ =\frac{30}{150}\\ =\frac{1}{5}\) There is an explanation video available below. |
|
| 46. |
Find the values of x and y respectively if A. -3, -2 B. -5, -3 C. -2, -5 D. -3, -5 Detailed Solution\(\begin{pmatrix} 1 & 0 \\ -1 & -1\\ 2 & 2 \end{pmatrix}\) + \(\begin{pmatrix} x & 1 \\ -1 & 0\\ y & -2 \end{pmatrix}\) = \(\begin{pmatrix} -2 & 1 \\ -2 & -1\\ -3 & 0 \end{pmatrix}\)therefore, (x, y) = (-3, -5) respectively There is an explanation video available below. |
|
| 47. |
\(\begin{pmatrix} -2 & 1 \\ 2 & 3 \end{pmatrix}\) \(\begin{pmatrix}p & q \\ r & s\end{pmatrix}\) = \(\begin{pmatrix} 1 & 0 \\0 & 1 \end{pmatrix}\). What is the value of r? A. -\(\frac{1}{8}\) B. \(\frac{3}{8}\) C. \(\frac{5}{8}\) D. \(\frac{1}{4}\) Detailed Solution-2p + r = 1.......(i)2p + 3r = 0.......(ii) r - 1 + 2p ........(iii) 2p + 3(1 + 2p) = 0 2p + 3(1 + 2p) = 0 2p + 3 + 6p = 0 3 - 8p = 0 \(\to\) 8p = 3 p = \(\frac{3}{8}\) 6 = 1 - 2 \(\frac{3}{8}\) = 1 - \(\frac{6}{8}\) \(\frac{8 - 6}{8}\) = \(\frac{2}{8}\) = \(\frac{1}{4}\) There is an explanation video available below. |
|
| 48. |
Calculate the distance between points L(-1, -6) and M(-3, -5) A. √5 B. 2√3 C. √20 D. √50 Detailed SolutionL\(\begin{pmatrix} x_1 & y_1 \\ -1 & -6 \end{pmatrix}\) m L\(\begin{pmatrix} x_2 & y_2 \\ -3 & -5 \end{pmatrix}\)D = \(\sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}\) D = \(\sqrt{(-3 - (-1)^2 + (-5 -(-6)^2}\) D = \(\sqrt{(-3 + 1)^2 + (-5 + 6)^2}\) D = \(\sqrt{(-2)^2 + 1^2}\) D = \(\sqrt{4 + 1}\) D = \(\sqrt{5}\) There is an explanation video available below. |
|
| 49. |
A student sitting on a tower 68 metres high observes his principal's car at the angle of depression of 20o. How far is the car from the bottom of the tower to the nearest metre? A. 184m B. 185m C. 186m D. 187m Detailed SolutionTan 20o = \(\frac{68m}{x}\)x tan 20o = 68 x = \(\frac{68}{tan 20}\) = \(\frac{68}{0.364}\) x = 186.8 = 187m There is an explanation video available below. |
| 41. |
Find the mean deviation of 2, 4, 5, and 9 A. 1 B. 2 C. 5 D. 7 Detailed Solution
= 5 mean deviation = 8/4 = 2 There is an explanation video available below. |
|
| 42. |
In how many ways can the letters of the word ACCEPTANCE be arranged? A. 10! / (2!2!3!) B. 10! / ( 2!3!) C. 10! / (2!2!) D. 10! Detailed SolutionACCEPTANCE = 10 LettersA = 2 letters C = 3 letters E = 2 letters Can be arranged in 10! / (2!3!2!) ways There is an explanation video available below. |
|
| 43. |
Find the number of ways of selecting 6 out of 10 subjects for an examination A. 128 B. 216 C. 215 D. 210 Detailed Solution\(^{10}C_6 = \frac{10!}{(10-6)!6!}\\=\frac{10!}{4!6!}\\ =\frac{10\times 9\times 8 \times 7 \times 6!}{4\times 3\times 2\times 1 \times 6!}\\ =210\) There is an explanation video available below. |
|
| 44. |
The probability of picking a letter T from the word OBSTRUCTION is? A. 1/11 B. 2/11 C. 3/11 D. 4/11 Detailed SolutionOBSTRUCTIONTotal possible outcome = 11 Number of chance of getting T = 2 P(picking T) = 2/11 There is an explanation video available below. |
|
| 45. |
 The result of rolling a fair die 150 times is as summarized in the table above. What is the probability of obtaining a 5? A. 3/10 B. 1/5 C. 1/6 D. 1/10 Detailed SolutionTotal possible outcome12+18+x+30+2x+45 = 105+3x ∴105+3x = 150 3x = 150-105 3x = 45 x = 15 P(obtaining 5) = \(\frac{2x}{(105+3x)}But x= 15\\ =\frac{2(15)}{(105+3(15))}\\ =\frac{30}{(105+45)}\\ =\frac{30}{150}\\ =\frac{1}{5}\) There is an explanation video available below. |
| 46. |
Find the values of x and y respectively if A. -3, -2 B. -5, -3 C. -2, -5 D. -3, -5 Detailed Solution\(\begin{pmatrix} 1 & 0 \\ -1 & -1\\ 2 & 2 \end{pmatrix}\) + \(\begin{pmatrix} x & 1 \\ -1 & 0\\ y & -2 \end{pmatrix}\) = \(\begin{pmatrix} -2 & 1 \\ -2 & -1\\ -3 & 0 \end{pmatrix}\)therefore, (x, y) = (-3, -5) respectively There is an explanation video available below. |
|
| 47. |
\(\begin{pmatrix} -2 & 1 \\ 2 & 3 \end{pmatrix}\) \(\begin{pmatrix}p & q \\ r & s\end{pmatrix}\) = \(\begin{pmatrix} 1 & 0 \\0 & 1 \end{pmatrix}\). What is the value of r? A. -\(\frac{1}{8}\) B. \(\frac{3}{8}\) C. \(\frac{5}{8}\) D. \(\frac{1}{4}\) Detailed Solution-2p + r = 1.......(i)2p + 3r = 0.......(ii) r - 1 + 2p ........(iii) 2p + 3(1 + 2p) = 0 2p + 3(1 + 2p) = 0 2p + 3 + 6p = 0 3 - 8p = 0 \(\to\) 8p = 3 p = \(\frac{3}{8}\) 6 = 1 - 2 \(\frac{3}{8}\) = 1 - \(\frac{6}{8}\) \(\frac{8 - 6}{8}\) = \(\frac{2}{8}\) = \(\frac{1}{4}\) There is an explanation video available below. |
|
| 48. |
Calculate the distance between points L(-1, -6) and M(-3, -5) A. √5 B. 2√3 C. √20 D. √50 Detailed SolutionL\(\begin{pmatrix} x_1 & y_1 \\ -1 & -6 \end{pmatrix}\) m L\(\begin{pmatrix} x_2 & y_2 \\ -3 & -5 \end{pmatrix}\)D = \(\sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}\) D = \(\sqrt{(-3 - (-1)^2 + (-5 -(-6)^2}\) D = \(\sqrt{(-3 + 1)^2 + (-5 + 6)^2}\) D = \(\sqrt{(-2)^2 + 1^2}\) D = \(\sqrt{4 + 1}\) D = \(\sqrt{5}\) There is an explanation video available below. |
|
| 49. |
A student sitting on a tower 68 metres high observes his principal's car at the angle of depression of 20o. How far is the car from the bottom of the tower to the nearest metre? A. 184m B. 185m C. 186m D. 187m Detailed SolutionTan 20o = \(\frac{68m}{x}\)x tan 20o = 68 x = \(\frac{68}{tan 20}\) = \(\frac{68}{0.364}\) x = 186.8 = 187m There is an explanation video available below. |