Year :
1996
Title :
Mathematics (Core)
Exam :
WASSCE/WAEC MAY/JUNE
Paper 1 | Objectives
1 - 10 of 49 Questions
| # | Question | Ans |
|---|---|---|
| 1. |
The 6th term of a G.P is \(\frac{-2}{27}\) and its first term is 18. What is the common ratio? A. -1/2 B. -1/3 C. 1/4 D. 2 E. 3 Detailed Solutiona, ar, ar\(^2\), ar\(^3\), ar\(^4\), ar\(^5\)6th term = ar\(^5\) = \(\frac{-2}{27}\) 1st term = a = 18 => ar\(^5\) = 18r\(^5\) =\(\frac{-2}{27}\) r\(^5\) = \(\frac{-2}{27 * 18}\) → \(\frac{-1}{27 * 9}\) r\(^5\) = \(\frac{-1}{243}\) r = \(^5\)√[\(\frac{-1}{243}\)] r = \(\frac{-1}{3}\) |
|
| 2. |
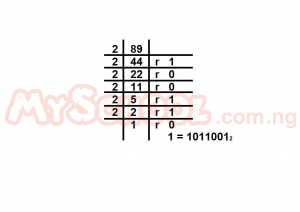
Convert the decimal number 89 to a binary number A. 101101 B. 111001 C. 1001001 D. 1001101 E. 1011001 Detailed Solution
|
|
| 3. |
Simplify: \((2\frac{1}{6} - 1\frac{2}{3}) \div 2\frac{2}{3}\). A. 3/16 B. 7/16 C. 1 13/24 D. 2 11/24 E. 10 2/5 Detailed Solution\((2\frac{1}{6} - 1\frac{2}{3}) \div 2\frac{2}{3}\)= \((\frac{13}{6} - \frac{5}{3}) \div \frac{8}{3}\) = \(\frac{3}{6} \times \frac{3}{8}\) = \(\frac{3}{16}\) |
|
| 4. |
Simplify 56x\(^{-4}\) \(\div\) 14x\(^{-8}\) A. 2x-12 B. 3x-3 C. 4x-4 D. 4x-3 E. 4x4 Detailed Solution\(56x^{-4} \div 14x^{-8}\)= \(4x^{- 4 - (-8)}\) = \(4x^{4}\) |
|
| 5. |
Simplufy Log104 + Log1025 A. 1 B. 2 C. 3 D. 4 E. 5 Detailed SolutionLog104 + Log1025 = Log10(4 + 25)= Log10100 = Log10102 = 2Log1010 = 2 x 1 = 2 |
|
| 6. |
A boy measured the length and breath of a rectangular lawn as 59.6m and 40.3m respectively instead of 60m and 40m. What is the percentage error in his calculation of the perimeter of the lawn? A. 10% B. 1.4% C. 7% D. 0.2% E. 0.1% Detailed SolutionLength = 59.6mBreath = 40.3m Perimeter = (59.6m + 40.3m) x 2 = 99.9 x 2 = 199.8m Actual measurement Perimeter = (60 + 40) x 2 = 100 x 2 = 200m Error = 200m - 199.8m = 0.2 Error =0.2/200 x 100/1 = 0.1% |
|
| 7. |
Find the 9th term of the arithmetic progression 18, 12, 6, 0, -6 ......... A. -54 B. -30 C. 30 D. 42 E. 66 Detailed Solution18, 12, 6, 0, -6, 12, -18, 24, -309th term = -30 |
|
| 8. |
Expand (2x - 5)(x - 3) A. x2 - 1x -15 B. 2x2 - 11x + 15 C. 22 - 5x - 8 D. x2 - 5x - 15 E. 2x2 - 6x + 15 Detailed Solution(2x - 5)(x - 3)2x\(^2\) - 5x - 6x + 15 2x\(^2\) - 11x + 15 |
|
| 9. |
Simplify: \(\frac{5}{x - y} - \frac{4}{y - x}\) A. 9x - y B. 9y - x C. 1x - y D. 1y - x E. y - 9xx - y Detailed Solution\(\frac{5}{x - y} - \frac{4}{y - x}\)= \(\frac{5(y - x) - 4(x - y)}{(x - y)(y - x)}\) = \(\frac{5y - 5x - 4x + 4y}{(x - y)(y - x)}\) = \(\frac{9y - 9x}{(x - y)(y - x)}\) = \(\frac{9(y - x)}{(x - y)(y - x)}\) = \(\frac{9}{x - y}\) |
|
| 10. |
If 3p - q = 6 and 2p + 3q = 4, find q A. o B. 1/2 C. 2/3 D. 1 E. 33/7 Detailed Solution3p - q = 6 ........ 12p + 3q = 4 ....... 2 Multiply eqn 1 by 3 9p - 3q = 18 ........ 3 2p - 3q = 4 ......... 2 Add eqn3 and eqn2 11p = 22 => p = 22/11 = 2 Substitute for p =2 in eqn1 3p - q = 6 3x2 - q = 6 => 6 - q = 6 => -q = 6 - 6 q = 0 |
| 1. |
The 6th term of a G.P is \(\frac{-2}{27}\) and its first term is 18. What is the common ratio? A. -1/2 B. -1/3 C. 1/4 D. 2 E. 3 Detailed Solutiona, ar, ar\(^2\), ar\(^3\), ar\(^4\), ar\(^5\)6th term = ar\(^5\) = \(\frac{-2}{27}\) 1st term = a = 18 => ar\(^5\) = 18r\(^5\) =\(\frac{-2}{27}\) r\(^5\) = \(\frac{-2}{27 * 18}\) → \(\frac{-1}{27 * 9}\) r\(^5\) = \(\frac{-1}{243}\) r = \(^5\)√[\(\frac{-1}{243}\)] r = \(\frac{-1}{3}\) |
|
| 2. |
Convert the decimal number 89 to a binary number A. 101101 B. 111001 C. 1001001 D. 1001101 E. 1011001 Detailed Solution
|
|
| 3. |
Simplify: \((2\frac{1}{6} - 1\frac{2}{3}) \div 2\frac{2}{3}\). A. 3/16 B. 7/16 C. 1 13/24 D. 2 11/24 E. 10 2/5 Detailed Solution\((2\frac{1}{6} - 1\frac{2}{3}) \div 2\frac{2}{3}\)= \((\frac{13}{6} - \frac{5}{3}) \div \frac{8}{3}\) = \(\frac{3}{6} \times \frac{3}{8}\) = \(\frac{3}{16}\) |
|
| 4. |
Simplify 56x\(^{-4}\) \(\div\) 14x\(^{-8}\) A. 2x-12 B. 3x-3 C. 4x-4 D. 4x-3 E. 4x4 Detailed Solution\(56x^{-4} \div 14x^{-8}\)= \(4x^{- 4 - (-8)}\) = \(4x^{4}\) |
|
| 5. |
Simplufy Log104 + Log1025 A. 1 B. 2 C. 3 D. 4 E. 5 Detailed SolutionLog104 + Log1025 = Log10(4 + 25)= Log10100 = Log10102 = 2Log1010 = 2 x 1 = 2 |
| 6. |
A boy measured the length and breath of a rectangular lawn as 59.6m and 40.3m respectively instead of 60m and 40m. What is the percentage error in his calculation of the perimeter of the lawn? A. 10% B. 1.4% C. 7% D. 0.2% E. 0.1% Detailed SolutionLength = 59.6mBreath = 40.3m Perimeter = (59.6m + 40.3m) x 2 = 99.9 x 2 = 199.8m Actual measurement Perimeter = (60 + 40) x 2 = 100 x 2 = 200m Error = 200m - 199.8m = 0.2 Error =0.2/200 x 100/1 = 0.1% |
|
| 7. |
Find the 9th term of the arithmetic progression 18, 12, 6, 0, -6 ......... A. -54 B. -30 C. 30 D. 42 E. 66 Detailed Solution18, 12, 6, 0, -6, 12, -18, 24, -309th term = -30 |
|
| 8. |
Expand (2x - 5)(x - 3) A. x2 - 1x -15 B. 2x2 - 11x + 15 C. 22 - 5x - 8 D. x2 - 5x - 15 E. 2x2 - 6x + 15 Detailed Solution(2x - 5)(x - 3)2x\(^2\) - 5x - 6x + 15 2x\(^2\) - 11x + 15 |
|
| 9. |
Simplify: \(\frac{5}{x - y} - \frac{4}{y - x}\) A. 9x - y B. 9y - x C. 1x - y D. 1y - x E. y - 9xx - y Detailed Solution\(\frac{5}{x - y} - \frac{4}{y - x}\)= \(\frac{5(y - x) - 4(x - y)}{(x - y)(y - x)}\) = \(\frac{5y - 5x - 4x + 4y}{(x - y)(y - x)}\) = \(\frac{9y - 9x}{(x - y)(y - x)}\) = \(\frac{9(y - x)}{(x - y)(y - x)}\) = \(\frac{9}{x - y}\) |
|
| 10. |
If 3p - q = 6 and 2p + 3q = 4, find q A. o B. 1/2 C. 2/3 D. 1 E. 33/7 Detailed Solution3p - q = 6 ........ 12p + 3q = 4 ....... 2 Multiply eqn 1 by 3 9p - 3q = 18 ........ 3 2p - 3q = 4 ......... 2 Add eqn3 and eqn2 11p = 22 => p = 22/11 = 2 Substitute for p =2 in eqn1 3p - q = 6 3x2 - q = 6 => 6 - q = 6 => -q = 6 - 6 q = 0 |